Next: 6 Sim(3): Similarity Transformations Up: 5 SE(2): Rigid transformations Previous: 5.2 Exponential Map
The adjoint in
![]() is computed from the generators:
is computed from the generators:
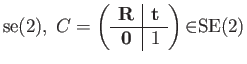 |
(129) | ||
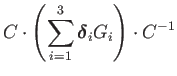 |
(130) | ||
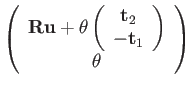 |
(131) | ||
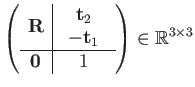 |
(132) |
Note that moving a tangent vector via the adjoint mixes the rotation component into the translation component.